Signals and Systems Important Questions
UNIT I
REPRESENTATION OF SIGNALS
PART-A (2 Marks)
1. Define Signal.
2. Define system.
3. What are the major classifications of the signal?
4. Define discrete time signals and classify them.
5. Define continuous time signals and classify them.
6. Define discrete time unit step &unit impulse.
7. Define continuous time unit step and unit impulse.
8. Define unit ramp signal.
9. Define periodic signal and non-periodic signal.
10. Define even and odd signal ?
11. Define Energy and power signal.
12. Define unit pulse function.
13. Define continuous time complex exponential signal.
14. What is continuous time real exponential signal.
15. What is continuous time growing exponential signal?
16. State the BIBO criterion for stability.
17. Find whether the signal given by x (n) = 5cos (6 _n) is periodic
18. Write down the exponential form of the Fourier series representation of a
Periodic signal?
19. Write down the trigonometric form of the fourier series representation of a
periodic signal?
20. Write short notes on dirichlets conditions for fourier series.
21. State Time Shifting property in relation to fourier series.
22. State parseval’s theorem for continuous time periodic signals.
PART – B
1. (a) For the systems represented by the following functions. Determine whether
every system is (1) stable (2) Causal (3) linear (4) Shift invariant (4)
(i) T[x(n)]= ex(n)
(ii) T[x(n)]=ax(n)+6
2. Determine whether the following systems are static or Dynamic, Linear or Nonlinear,Shift variant or Invarient, Causal or Non-causal, Stable or unstable. (4)
(i) y(t) = x(t+10) + x2(t)
(ii) dy(t)/dt + 10 y(t) = x(t)
3. Explain about the properties of continuous time fourier series. (8)
4. Find the fourier coefficients of the given signal. (4)
x(t) = 1+ sin 2_ot + 2 cos 2_ot + cos (3_ot + _/3)
5. Determine the Fourier series coefficient of exponential representation of x(t)
x(t) = 1, ItI (8)
0, T1< ItI < T/ 2
6. Find the exponential series of the following signal. (8)
7. Find which of the following signal are energy or power signals. (8)
a) x(t)=e-3t u(t) b) x(t) = ej(2t+_/4) c) x(n)= cos(_/4n)
8. Explain the properties of Discrete time fourier serier (8)
9. Find the cosine fourier series of an half wave rectified sine function. (8)
10. Explain the classification of signals with examples. (8)
UNIT II ANALYSIS OF CONTINUOUS TIME SIGNALS AND SYSTEMS
PART-A (2 Marks)
1. Define continuous time system.
2. Define Fourier transform pair.
3. Write short notes on dirichlets conditions for fourier transform.
4. Explain how aperiodic signals can be represented by fourier transform.
5. State convolution property in relation to fourier transform.
6. State parseval’s relation for continuous time fourier transform.
7. What is the use of Laplace transform?
8. What are the types of laplace transform?
9. Define Bilateral and unilateral laplace transform.
10. Define inverse laplace transform.
11. State the linearity property for laplace transform.
12. State the time shifting property for laplace transform.
13. Region of convergence of the laplace transform.
14. What is pole zero plot.
15. State initial value theorem and final value theorem for laplace transform.
16. State Convolution property.
17. Define a causal system.
18. What is meant by linear system?
19. Define time invariant system.
20. Define stable system?
21. Define memory and memoryless system.
22. Define invertible system.
23. What is superposition property?
24. Find the fourier transform of x(t)=cos(_0t)
PART – B
1. Determine the inverse laplace of the following functions. (6)
1) 1/s(s+1) 2) 3s2 +8s+6 (s+2)(s2+2s+1)
2. Explain about the classifications of continuous time system. (8)
3. A system is described by the differential equation. (10) d2y(t)/dt2+3dy(t)/dt+2y(t)=dx(t)/dt if y(0) =2;dy(0)/dt = 1 and x(t)=e-t u(t) Use laplace transform to determine the response of the system to a unit step input applied at t=0.
4. Obtain the transfer function of the system when y(t) = e-t-2 e-2t+ e-3t and x(t)= e-0.5t (8) 5. a) Discuss the condition on stability of an LTI system based on Laplace domain representation. (3)
b) Bring the equivalence between Laplace transform and Fourier transform.(5)
6. Explain the properties of laplace transform (8)
7. Find the impulse and step response of the following systems H(s) = 10/s2+6s+10 (6)
8.For the transfer function H(s) = s+10/ s2+3s+2 find the response due to input x(t) = sin2(t) u(t) (6)
9. Find the fourier transform of triangular pulse (10) x(t) = _(t/m) ={1-2|t|/m |t| 0 otherwise
10. The input and output of a causal LTI system are related by the differential equation. (10)
d2y(t)/dt2+6dy(t)/dt+8y(t)=2x(t) i) Find the impulse response of the system. ii) What is the
response of this system if x(t) = t e-2t u(t) 11. Consider a causal LTI system with frequency
response. (10) H(j_) = 1/ j_ +2 For a particular input x(t) this system is y(t)= e-2t u(t) – e-3t u(t)
UNIT III SAMPLING THEOREM AND Z – TRANSFORMS
PART-A (2 Marks)
1. Why CT signals are represented by samples.
2. What is meant by sampling.
3. State Sampling theorem.
4. What is meant by aliasing.
5. What are the effects aliasing.
6. How the aliasing process is eliminated.
7. Define Nyquist rate.and Nyquist interval.
8. Define sampling of band pass signals.
9. Define Z transform.
10. What are the two types of Z transform?
11. Define unilateral Z transform.
13. What is region of Convergence.
14. What are the Properties of ROC.
15. What is the time shifting property of Z transform.
16. What is the differentiation property in Z domain.
17. State convolution property of Z transform.
18. State the methods to find inverse Z transform.
19. State multiplication property in relation to Z transform.
20. State parseval’s relation for Z transform.
21. What is the relationship between Z transform and fourier transform.
22. What is meant by step response of the DT system.
PART – B
1.State and prove the sampling theorem. Also explain how reconstruction of original signal is done from sampled signal (16)
2. Find the Z – transform of the signal (8) (i)x(n)= nan u(n) (ii)x(n)= an cos(_0) u(n)
3. Determine the inverse z transform of the following function x(z)=1/(1+z-1) (1-z-1 )2 ROC : |Z>1|
4. Explain the properties of z-transform (8)
5. Find the z-transform of x(z)= 1+2z-1 / 1- 2z-1 + z-2 if x(n) is anticausal using long
division method. (8)
6. find the inverse z-transform of x(z)= 1+3z-1 / 1+ 3z-1 + 2z-2 using residue method(8)
7. Give the relationship between z-transform and fourier transform. (8)
UNIT IV DISCRETE TIME SYSTEMS
PART-A (2 Marks)
1. Define Transfer function of the DT system.
2. Define impulse response of a DT system.
3. State the significance of difference equations.
4. Write the differece equation for Discrete time system.
5. Define frequency response of the DT system.
6. What is the condition for stable system.
7. What are the blocks used for block diagram representation.
8. State the significance of block diagram representation.
9. What are the properties of convolution?
10. State theCommutative properties of convolution?
11. State the Associative properties of convolution
12. State Distributive properties of convolution
13. Define causal system.
14. What is the impulse response of the system y(t)=x(t-t0).
15. What is the condition for causality if H(z) is given.
16. What is the condition for stability if H(z) is given.
17. Check whether the system is causal or not ,the H(z) is given by (z3 + z)/(z+1).
18. Check whether the system is stable or not ,the H(z) is given by (z/z-a).,|a|<1.
19. Determine the transfer function for the sys tem described by the difference
equation y(n)- y(n-1) = x(n)- x(n-2).
20. How the discrete time system is represented.
PART – B
1. Give the properties of convolution (6)
2. Determine the step response of the difference equation, y(n)-(1/9)y(n-2)=x(n-1)
with y(-1)=1 and y(-2)=0 (8)
3. Find the impulse response and step response.
Y(n)-3/4y(n-1) +1/8 y(n-2) = x(n) (8)
4. Find the output y(n) of a linear time invariant discrete time system specified by the
equation. (16)
Y(n)-3/2y(n-1) +1/2 y(n-2) = 2x(n) +3/2 x(n-1) when initial conditions are y(-1)
=0,y(-2) = 1 and input x(n)=(1/4)n u(n)
5. Determine the Nyquist sampling rate and Nyquist sampling intervals for
sinc(200_t) + 3sinc2(120_t) (6)
6. Find the frequency response of the following causal system.
Y(n)=1/2x(n)+x(n-1)+1/2 x(n-2) (4)
7. Determine inverse Discrete Time Fourier Transform of
X(k)={1,0,1,0} (8)
8. Give the summary of elementary blocks used to represent discrete (4)
time systems.
UNIT V SYSTEM WITH FINITE AND INFINITE DURATION IMPULSE RESPONSE
PART-A (2 Marks)
1. What is meant by FIR system.
2. What is meant by IIR system.
3. What is recursive system?
4. What is Non recursive system?
5. What is the difference between recursive and non recursive system
6. Define realization structure.
7. What are the different types of structure realization.
8. What is natural response?
9. What is zero input Response?
10. What is forced response?
11. What is complete response?
12. Give the direct form I structure.
13. Give the direct form II structure..
14. How the Cascade realization structure obtained.
15. Give the parallel for Realization structure.
16. What is transformed structure representation?
PART – B
1..a) Determine the transposed structure for the system given by difference equation
y(n)=(1/2)y(n-1)-(1/4)y(n-2)+x(n)+x(n-1) (16)
b) Realize H(s)=s(s+2)/(s+1)(s+3)(s+4) in cascade form
2. A difference equation of a discrete time system is given below:
y(n)-3/4 y(n-1) +1/8 y(n-1) = x(n) +1/2 x(n-1)
draw direct form I and direct form II. (6)
3. Realize the following structure in direct form II and direct form I
H(s) = s+1/s2 + 3s+5 (10)
4. Determine the recursive and nonrecursive system (16)
5. Determine the parallel form realization of the discrete time system is
y(n) -1/4y(n-1) -1/8 y(n-2) = x(n) +3x(n-1)+2x(n-2) (10)







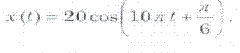







 0
0 =25 nc/m lies on the line, x=-3m and y=4m, in free space. Find the electric field intensity at a point(2,3,15)m
=25 nc/m lies on the line, x=-3m and y=4m, in free space. Find the electric field intensity at a point(2,3,15)m
